Polyhedron geometry definition
WebApr 6, 2024 · Polyhedron definition states that “a three-dimensional structure in Euclidean geometry, made up of a finite number of polygonal faces”. The boundary between the interior and the exterior of a solid is a polyhedron. Polyhedrons, in general, are named according to the number of faces. Parts of Polyhedron. The Polyhedron has three parts … WebPolyhedron Definition. A three-dimensional shape with flat polygonal faces, straight edges, and sharp corners or vertices is called a polyhedron. Common examples are cubes, prisms, pyramids. However, cones, and spheres are not polyhedrons since they do not have … In geometry, a rectilinear figure can be defined as a plane figure or shape all of … Real-Life Examples of a Parallelogram. When we look around us, we can see … How to Find Prime Numbers. In the third century BCE, the Greek mathematician … Fraction of a Whole. When the whole is divided into equal parts, the number of … The basic definition of multiple is manifold. In math, the meaning of a multiple is the … What Is a Factor? A factor is a number that divides another number, leaving no … What is Place Value? In math, every digit in a number has a place value. Place value … Geometry. PreK-5. Progression of Topics in Online Math Games for Kids. Let’s have a …
Polyhedron geometry definition
Did you know?
WebThe term ‘coordination polyhedron’ describes ligands’ geometric pattern or spatial arrangement directly linked to the centre atom or an ion. The most frequent coordination polyhedra are tetrahedral, octahedral, and square planar geometries. For example: The geometry of [Ni(CO)4] is tetrahedral; The geometry of [Ni(CN)4]2- is square planar WebMar 28, 2024 · Definition. A hexagon is a polygon having six straight sides and six angles. The word ‘hexagon’ came from the Greek word ‘hex’ meaning ‘six’ and ‘gonia’ meaning ‘corner, angle’. When all the six sides and angles of a hexagon are equal, it is called a regular hexagon. Otherwise, it is an irregular hexagon.
WebPolyhedrons. A polyhedron is a 3-dimensional figure that is formed by polygons that enclose a region in space. Each polygon in a polyhedron is called a face. The line segment where two faces intersect is called an edge and the point of intersection of two edges is a vertex. There are no gaps between the edges or vertices in a polyhedron. Web1 day ago · It is obvious that the answer is [1,0], [0,1], [0,0]. I only need this basic example to understand how pycddlib works for more advanced tasks. The pycddlib documentation and code examples at this website like this one ( Polytope, Python - find extreme points) use only one matrix to define the polyhedron. It is clear that this matrix must be ...
WebA tessellation of simple polyhedra is easy to imagine from the case of one isolated polyhedron, and the corresponding conceptual model (Figure 10.21), is different only with respect to certain cardinalities.That is, edges are part of two or more facets, rather than just one, and a facet can be part of two or more polyhedra, rather than just one. WebMar 29, 2016 · Algorithms for skyline querying based on wireless sensor networks (WSNs) have been widely used in the field of environmental monitoring. Because of the multi-dimensional nature of the problem of monitoring spatial position, traditional skyline query strategies cause enormous computational costs and energy consumption. To ensure the …
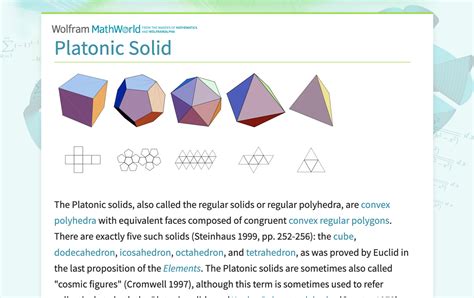
http://www.mathematicsmagazine.com/Articles/SacredGeometryPlatonicSolids.php
WebJul 20, 2024 · A polyhedron (plural: polyhedra) is a closed geometric shape made entirely of polygonal sides.; A face is a polygonal side of a polyhedron.; An edge is a line segment where two faces meet.; A vertex, or corner, is a point where two or more edges meet.; A polyhedron is regular if all the faces are regular polygons and are congruent to each other … small travel golf bagWebA solid with flat faces. Each flat face is a polygon. Polyhedron comes from Greek poly- meaning "many" and -hedron meaning "face". Examples include prisms, pyramids, cubes and many more. See: Polygon. hiit athleticWebpolyhedron, In Euclidean geometry, a three-dimensional object composed of a finite number of polygonal surfaces (faces). Technically, a polyhedron is the boundary between the interior and exterior of a solid. In general, polyhedrons are named according to number of faces. A tetrahedron has four faces, a pentahedron five, and so on; a cube is a six-sided regular … hiit backWebFirst we must take into account the following in order to calculate the area, volume and radius of the regular polyhedrons: A = A = area. V = V = volume. a = a = edge. R = R = radius of the circumscribed sphere. r = r = radius of the inscribed sphere. \rho = ρ = radius of the sphere tangent to the edges. hiit at the gymWebA choice of ω is a linear function on R n which restricts to a linear function on the polyhedron. The face of ω is the locus of points of the polyhedron maximizing the function (exists by compactness yadda yadda), which is going to be one of the lower-dimensional cells. *at least one of (only one, if the polyhedron is convex). small travel humidifier walmartWebWalter Meyer, in Geometry and Its Applications (Second Edition), 2006. DEFINITION. A polyhedron is a three-dimensional solid bounded by a finite number of polygons called faces. Points where three or more faces meet are called vertices. Line segments where exactly two faces meet at an angle are called edges. The vertices and edges of the … hiit back and bicep workoutWebTherefore, a polyhedron comprises three kinds of geometric objects - vertices, edges and faces. Definition 6. A polyhedron is said to be regular if all its faces are equal regular polygons and the same number of faces meet at every vertex. A polyhedron formed by the {p} polygons with q meeting at every vertex is denoted {p, q}. hiit at planet fitness